- Scilabヘルプ
- CACSD
- Formal representations and conversions
- Plot and display
- abinv
- arhnk
- arl2
- arma
- arma2p
- arma2ss
- armac
- armax
- armax1
- arsimul
- augment
- balreal
- bilin
- bstap
- cainv
- calfrq
- canon
- ccontrg
- cls2dls
- colinout
- colregul
- cont_mat
- contr
- contrss
- copfac
- csim
- ctr_gram
- damp
- dcf
- ddp
- dhinf
- dhnorm
- dscr
- dsimul
- dt_ility
- dtsi
- equil
- equil1
- feedback
- findABCD
- findAC
- findBD
- findBDK
- findR
- findx0BD
- flts
- fourplan
- freq
- freson
- fspec
- fspecg
- fstabst
- g_margin
- gamitg
- gcare
- gfare
- gfrancis
- gtild
- h2norm
- h_cl
- h_inf
- h_inf_st
- h_norm
- hankelsv
- hinf
- imrep2ss
- inistate
- invsyslin
- kpure
- krac2
- lcf
- leqr
- lft
- lin
- linf
- linfn
- linmeq
- lqe
- lqg
- lqg2stan
- lqg_ltr
- lqr
- ltitr
- macglov
- minreal
- minss
- mucomp
- narsimul
- nehari
- noisegen
- nyquistfrequencybounds
- obs_gram
- obscont
- observer
- obsv_mat
- obsvss
- p_margin
- parrot
- pfss
- phasemag
- plzr
- pol2des
- ppol
- prbs_a
- projsl
- repfreq
- ric_desc
- ricc
- riccati
- routh_t
- rowinout
- rowregul
- rtitr
- sensi
- sident
- sorder
- specfact
- ssprint
- st_ility
- stabil
- sysfact
- syslin
- syssize
- time_id
- trzeros
- ui_observer
- unobs
- zeropen
Please note that the recommended version of Scilab is 2026.0.0. This page might be outdated.
See the recommended documentation of this function
arl2
L2伝達関数近似による SISO モデル実現
呼び出し手順
h=arl2(y,den0,n [,imp]) h=arl2(y,den0,n [,imp],'all') [den,num,err]=arl2(y,den0,n [,imp]) [den,num,err]=arl2(y,den0,n [,imp],'all')
引数
- y
z^-1の実数ベクトルまたは多項式で, 有理数システムの(インパルス応答の)フーリエ級数近似の係数を有します.- den0
解の初期推定値を指定する多項式で,
poly(1,'z','c')とすることができます.- n
整数, 伝達関数近似の次数 (分母denの次数)
- imp
整数で値は
(0,1,2)のどれか (冗長モード)- h
伝達関数
num/denまたは'all'フラグが指定された場合は伝達行列 (列ベクトル)'all'.- den
多項式または多項式ベクトル,解の分母
- num
多項式または多項式ベクトル, 解の分子
- err
実数の定数またはベクトル , 各解のL2誤差
説明
[den,num,err]=arl2(y,den0,n [,imp]) は,
伝達関数num/denが安定で,そのインパルス応答が
無数のゼロ点で完結すると仮定したベクトル y を
(最小L2ノルムで)近似する
多項式対num および denを探します.
y(z) = y(1)(1/z)+y(2)(1/z^2)+ ...+ y(ny)(1/z^ny)の場合,
num/den - y(z)のL2ノルムは,errとなります.
n は多項式 denの次数です.
伝達関数num/denは,有理システムのフーリエ級数のL2近似です.
impの設定により様々な中間結果が出力されるようになります.
[den,num,err]=arl2(y,den0,n [,imp],'all') は,
多項式のベクトルnum および denに
問題のローカル最適解の集合を返します.
解は誤差について昇順にソートされます.
この場合, den0はすでに
poly(1,'z','c')であると仮定されます.
例
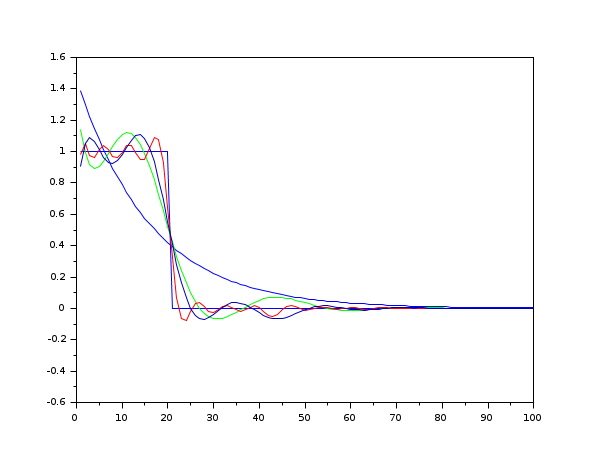
v=ones(1,20); clf(); plot2d1('enn',0,[v';zeros(80,1)],2,'051',' ',[1,-0.5,100,1.5]) [d,n,e]=arl2(v,poly(1,'z','c'),1) plot2d1('enn',0,ldiv(n,d,100),2,'000') [d,n,e]=arl2(v,d,3) plot2d1('enn',0,ldiv(n,d,100),3,'000') [d,n,e]=arl2(v,d,8) plot2d1('enn',0,ldiv(n,d,100),5,'000') [d,n,e]=arl2(v,poly(1,'z','c'),4,'all') plot2d1('enn',0,ldiv(n(1),d(1),100),10,'000')
参照
| Report an issue | ||
| << arhnk | CACSD | arma >> |