Please note that the recommended version of Scilab is 2026.0.0. This page might be outdated.
See the recommended documentation of this function
legendre
associated Legendre functions
Calling Sequence
y = legendre(n,m,x [,normflag])
Arguments
- n
non negative integer or vector of non negative integers regularly spaced with increment equal to 1
- m
non negative integer or vector of non negative integers regularly spaced with increment equal to 1
- x
real matrix(elements of
xmust be in the[-1,1]interval)- normflag
(optional) scalar string
Description
When n and m are scalars,
legendre(n,m,x) evaluates the associated Legendre
function Pnm(x) at all the elements of x. The
definition used is :
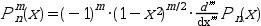
where Pn is the Legendre polynomial of degree
n. So legendre(n,0,x) evaluates the
Legendre polynomial Pn(x) at all the elements of
x.
When the normflag is equal to "norm" you get a normalized version
(without the (-1)^m factor), precisely :
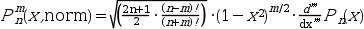
which is useful to compute spherical harmonic functions (see Example 3):
For efficiency, one of the two first arguments may be a vector, for
instance legendre(n1:n2,0,x) evaluates all the Legendre
polynomials of degree n1, n1+1, ..., n2 at the
elements of x and
legendre(n,m1:m2,x) evaluates all the Legendre
associated functions Pnm for m=m1, m1+1, ..., m2 at
x.
Output format
In any case, the format of y is :
and :
y(i,j) = P(n(i),m;x(j)) if n is a vector y(i,j) = P(n,m(i);x(j)) if m is a vector y(1,j) = P(n,m;x(j)) if both n and m are scalars
so that x is preferably a row vector but any
mx x nx matrix is expected and considered as an
1 x (mx * nx) matrix, reshaped following the column
order.
Examples
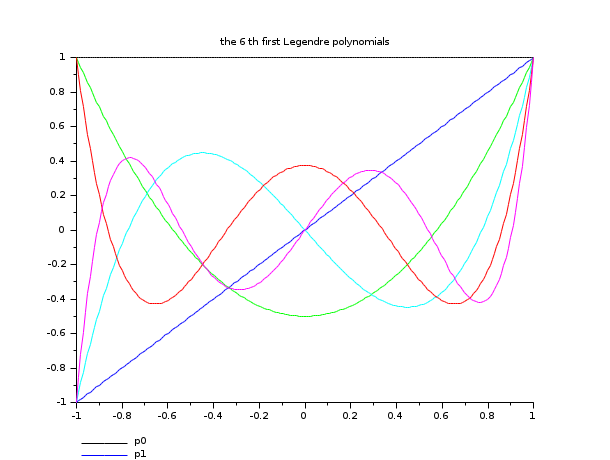
// example 1 : plot of the 6 first Legendre polynomials on (-1,1) l = nearfloat("pred",1); x = linspace(-l,l,200)'; y = legendre(0:5, 0, x); clf() plot2d(x,y', leg="p0@p1@p2@p3@p4@p5@p6") xtitle("the 6 th first Legendre polynomials")
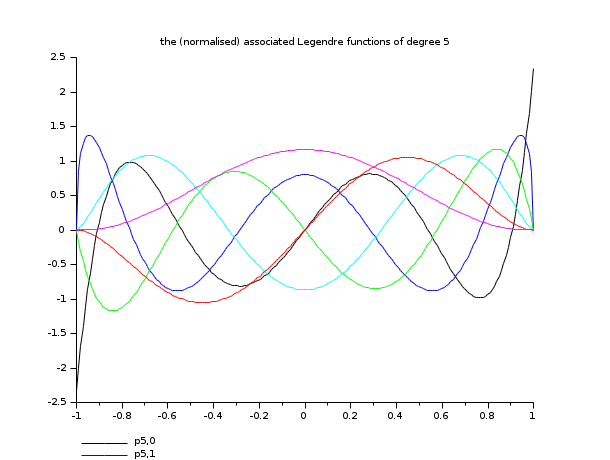
// example 2 : plot of the associated Legendre functions of degree 5 l = nearfloat("pred",1); x = linspace(-l,l,200)'; y = legendre(5, 0:5, x, "norm"); clf() plot2d(x,y', leg="p5,0@p5,1@p5,2@p5,3@p5,4@p5,5") xtitle("the (normalized) associated Legendre functions of degree 5")
// example 3 : define then plot a spherical harmonic // 3-1 : define the function Ylm function [y]=Y(l, m, theta, phi) // theta may be a scalar or a row vector // phi may be a scalar or a column vector if m >= 0 then y = (-1)^m/(sqrt(2*%pi))*exp(%i*m*phi)*legendre(l, m, cos(theta), "norm") else y = 1/(sqrt(2*%pi))*exp(%i*m*phi)*legendre(l, -m, cos(theta), "norm") end endfunction // 3.2 : define another useful function function [x, y, z]=sph2cart(theta, phi, r) // theta row vector 1 x nt // phi column vector np x 1 // r scalar or np x nt matrix (r(i,j) the length at phi(i) theta(j)) x = r.*(cos(phi)*sin(theta)); y = r.*(sin(phi)*sin(theta)); z = r.*(ones(phi)*cos(theta)); endfunction // 3-3 plot Y31(theta,phi) l = 3; m = 1; theta = linspace(0.1,%pi-0.1,60); phi = linspace(0,2*%pi,120)'; f = Y(l,m,theta,phi); [x1,y1,z1] = sph2cart(theta,phi,abs(f)); [xf1,yf1,zf1] = nf3d(x1,y1,z1); [x2,y2,z2] = sph2cart(theta,phi,abs(real(f))); [xf2,yf2,zf2] = nf3d(x2,y2,z2); [x3,y3,z3] = sph2cart(theta,phi,abs(imag(f))); [xf3,yf3,zf3] = nf3d(x3,y3,z3); clf() subplot(1,3,1) plot3d(xf1,yf1,zf1,flag=[2 4 4]); xtitle("|Y31(theta,phi)|") subplot(1,3,2) plot3d(xf2,yf2,zf2,flag=[2 4 4]); xtitle("|Real(Y31(theta,phi))|") subplot(1,3,3) plot3d(xf3,yf3,zf3,flag=[2 4 4]); xtitle("|Imag(Y31(theta,phi))|")
| Report an issue | ||
| << gamma | Fonctions spéciales | %asn >> |